25. The Colourful Hat Problem

…because everything’s better with zombies!

You are the general in charge of an army division which has been assigned the task of clearing an island of zombies. To make the job easier, you’ve divided it into 21 zones and assigned one unit of soldiers to clear each zone. The plans are all in place and the soldier are raring to go. Before you give the order, you check everything over one last time. That’s when you realise there’s as a problem: where one zone meets another, there’s a risk of soldiers from different units getting mixed up if they run into each other, especially it’s during a battle with a zombie horde. This could leave some of the units dangerously under-manned and unable to continue with their assignment until they get their missing men back. This means such confusion will slow the whole mission down, and could even result in its failure. What you need, you realise, is an easy way for the soldiers to tell which men are in which units.

Suddenly, the solution comes to you: since zombies only react to sound and movement rather than colour so there’s really no need for camouflage. This means you can simply provide brightly coloured hats to each unit, and make sure that units assigned to neighbouring zones are given different coloured hats. However, the more different coloured hats you need to order the longer it will take to start the mission, and the more zombies there will be when the fighting starts, making it more dangerous and difficult, so speed is of the essence. Given the zones you have created, what’s the minimum number of different colours of hat would you need so that the soldiers in every zone can be assigned a colour of hat that’s different from those being used in all neighbouring zones?

A: 2.

B: 3.

C: 4.

D: 5.

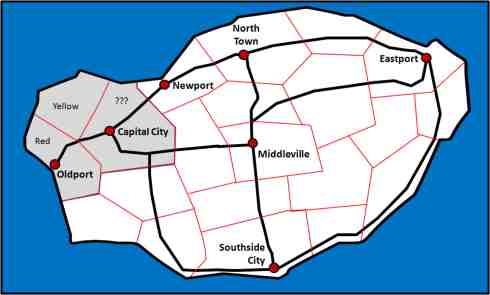
The Map Of The Island Showing The 21 Zones (Marked In Red):

Scroll down to see the right answer…

What answer did you get?

A: Whoa, that’s not nearly enough different colours, there will be confusion all over the place and your poor choice has put the whole mission in danger.

B: Three colours will work for most of the zones, but there will still be the potential for confusion in the area around zone 17 because two neighbouring zone will have to contain soldiers with hats of the same colour. This probably won’t endanger the whole mission, but it might slow things down.

C: Spot on. With four different colours of hats you can assign every unit a hat colour that will be different from the ones worn in every neighbouring zone.

D: That’s too many, and you’ll have wasted precious time getting hats in a colour that you don’t need. Let’s hope the zombies haven’t multiplied in this time to levels that your men can’t handle.

How to work it out: The key to solving this problem is to use something called ‘Minimal Criminals’. For this problem, a minimal criminal is the smallest example of a group of zones which shows that you cannot use a given number of colours to successfully assign different hat colours to all neighbouring zones. If you find even one of these minimal criminals on your map for a given number of colours then you cannot successfully assign the coloured hats to each zone.

Here’s an example of a minimal criminal which shows that you cannot use just two colour of hats. If you assign the red to zone 2, and yellow to zone 1, then you’d have to use a third colour for zone 4 since it touches both of these zones. There’s lots of these minimal criminals on this map.

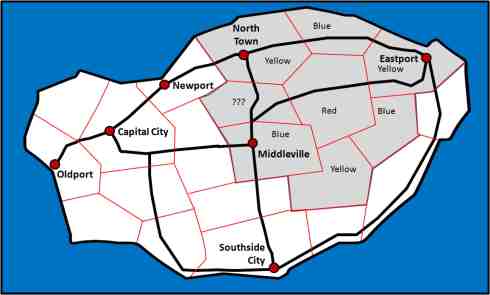
A minimal criminal which shows that you cannot use just three colours is harder to find, but there is one, and it’s shaded in grey on the map below. This consists of a central zone surrounded by seven other zones. Each of these surrounding zones touches the central zone so they must have different colours to it. If the zones surrounding it are then assigned alternating colours, you then come to a situation with the final zone because it touches the central zone and zones with each of the two other colours. This means it has to be given a fourth colour.

Since there’s no minimal criminals which mean you can’t use four colours, you know that four different coloured hats will be enough. In fact, it’s impossible to divide the island up into zones which would require more than four colours, and this is true of any map. This is known as the four colour theorem, and while it was proposed many years ago, it was not proven until 1976 and was the first mathematical theorem which was solved using a computer.

If you want to find out more about the four colour theorem (including the rules for creating the maps and deciding which zones are considered neighbours), click here.

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

24. The Six Bridges Problem

…because everything’s better with zombies!

You’re holidaying on a small archipelago of islands when a zombie outbreak catches you unaware. You manage to get to a safe house, but there’s no supplies of any kind and this means you’ll need to go on a supply run. You look at a map (see below) of the five islands in the archipelago and see that they’re connected by six bridges. Your safe house is on the northern-most island and you can see that there’s a warehouse where you can get food and drink from on the large island to the southwest. There’s a hospital where you’ll be able to get medical supplies on another large island to the southeast. Between these two large islands are a couple of small ones, one of which has a gun store on it, where you will be able to get weapons, while the other has an ammunition dump on it where you can get plenty of ammunition. You look out the window and see the zombies shambling around. They look slow and you know you’d probably be able to out-run them, but the moment they know you’re there, they’ll start following you. This means that once you cross a bridge, they’ll follow you onto it and you won’t be able to cross it again because there will be too many zombies on it. Is there a route, starting from your safe house, which will allow you to visit all the places you want to go for supplies and make it back to your safe house without having to use any of the bridges twice?

A: Yes, there’s a route which allows me to visit all the places need to go to get supplies and still get back to the safe house without having to use any of the bridges twice. I’m setting off now before things get any worse.

B: There’s no route which will allow me to visit everywhere I need to go and still get back to the safe house without having to cross the same bridge twice. The most places I can visit is three out of the four, so I’ll need to decide which one to leave out on my supply run.

Scroll down to see the right answer…

A: You’re wrong, there’s no route which will allow you to visit all four places and make it back to the safe house without having to use one of the bridges twice. If you set out and try to do it, you’ll end up in big trouble!

B: You’re right, there’s no route which will allow you to get to all four locations without crossing a bridge twice. The only decision left now is which place to leave out.

How to work it out: There’s two ways to solve this problem. One is trial and error, where you try every single possible route, but that will take time, and in a zombie apocalypse speed is of the essence if you want to survive. The other is to think about it from a mathematical perspective. Each island has one place you want to visit on it. This means that you’ll need to be able to reach each island once and leave it once (otherwise you can’t get back to where you started). If you ever go onto an island and can’t leave it by a bridge you haven’t already used, you’ll be stuck. Because you have to cross every bridge on the map at least once to visit all the places you need to go, this means that if any island is connected to other islands by an odd number of bridges, you’ll end up getting stuck on it at some point. So to work out whether it is ever possible to visit every island without to using any bridge twice, all you need to do is see whether any islands are connected by an odd number of bridges. In this case, there’s two of them, the big ones to the southeast and the southwest, so if you know your maths, you can know instantly that there’s no safe route which will allow you to visit everywhere and still get back to the safe house without crossing a zombie-infested bridge, and that wouldn’t be a very good idea.

If you want to explore this problem further, you can try adding another bridge and see how this changes the outcome. If you do this, you’ll see that no matter which pair of islands you connect with a new bridge, you can manage to get round all four intended destinations and back to your safe house without having to cross any bridge twice. Why does this make a difference? It’s because this means you no longer need to use every bridge to complete your trip so the rule about needing an even number of bridges attached to each island no longer applies.

If you dig into this even deeper, you’ll see that with any number of bridges of seven and above, as long as each island has at least two bridges connecting it to other islands (i.e. so there’s no dead ends), you can complete your intended circuit safely, while any number of bridges of five of fewer, there will be at least one island only connected to another by a single bridge, meaning you can’t do it. Six is the only number of bridges which allows you to have no dead ends, but still not be able to complete your supply run successfully, and this only works with some placements of bridges and not others. For example, if the upper small island was connected to the big island to its north as its second bridge rather than the one to the southwest, you’d be able to complete the journey successfully. Again, this is because you no longer have to use all the bridges.

For those who are interested, this problem is based on a rather old puzzle called the Königsberg Bridge problem. If you want to find out more about this original problem, click here. This was the first mathematical problem every solved with graph theory, and its original solution by Euler laid the foundations for topology. This makes the Königsberg Bridge problem an important landmark in the history of mathematics.

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

23. The Big Freeze Problem

…because everything’s better with zombies!

The zombie apocalypse has begun and a large group of you have sought refuge in the far North because you’ve heard that zombies can’t move at low temperatures. This is because they seize up if they get too cold. However, at the northern latitudes where you’re hiding out, it’s not always sufficiently cold for this to happen, and for three months of the year (June, July and August) the temperatures are warm enough for the zombie to advance. This is quickly becoming known as the walking season and is dreaded by everyone since it’s when almost all zombie attacks take place. Only 30% of the zombies survive their annual chill, and in each month when it’s warm enough for them to move, they can stagger 100 miles. It’s now the end of August and the temperatures have just started to drop so the zombies have frozen up for the winter meaning this year’s walking season is over. You’ve estimated that 2 million zombies crossed the Canadian border, heading north, just before this happened. How long will it take for this zombie horde to reach you 1,300 miles to the North and how many will be left when they do?

A: It will take four years for them to reach us, and there will be 16,200 zombies left when they do.

B: They’ll reach us in four years and ten months, and there will only be 4,860 left by then.

C: It will take the zombies five years and ten months to get here, and there will be 1,458 left.

D: All the zombies will die from the cold before they reach here so we’re completely safe.

Scroll down to see the right answer…

What answer did you get?

A: You’re almost right, but the zombies will still be 100 miles away after four years and they will have to survive another winter before they can cover these last few miles. This will thin out their numbers even more meaning there will be many fewer for you to worry about.

B: Spot on! Now you know when the zombies will arrive and how many there will be, you can start preparing for their arrival.

C: I don’t know what went wrong there, but you’re a few months out, and there will be many more zombies than you’re expecting. This means you’ll be caught unaware and unprepared when they finally turn up on your door step.

D: I don’t know what went wrong there, but you’re a few months out, and there will be many more zombies than you’re expecting. This means you’ll be caught unaware and unprepared when they finally turn up on your door step.

How to work it out: At the start of this problem, the 2 million zombies are frozen in place 1,300 miles to your south. This means they won’t be able to start moving until the temperatures warm again next June. During this time, only 30% (or 0.3, if we express this as a decimal fraction) will survive. To work out how many this is, multiply the initial number (2 million) by the percentage expressed as a decimal fraction (0.3). This calculation tells you that at the start of the next summer there will only be 600,000 zombies left. Over the next three months, they’ll stagger 100 miles each month, or 300 miles in total. So, in exactly one year’s time, when the zombies freeze up again, there will be a horde of 600,000 zombies 1,000 miles away (the initial 1,300 minus the 300 they’ve been able to move in the summer months). If you repeat these calculations for the next year, starting with these new values, you’ll find that at the end of the second year, the zombies will be 700 miles away and there will be 180,000 of them. At the end of the third year, there will be just 54,000 left and they’ll be 400 miles away. At the end of the fourth year, they’ll be only 100 miles away, but their numbers will have been whittled down to 16,200. During the next winter, 70% of these zombies will die, meaning there will only be 4,860 still alive to start walking nine months later at the beginning of the following June. Since they can cover 100 miles per month, this means these remaining zombies will reach you at the start of July. So in total, you’ve got four years and ten months to work out a way for you to be able to kill almost 5,000 zombies before any of them get you. Got any ideas?

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

22. The Recruitment Problem

…because everything’s better with zombies!

There’s a zombie outbreak in a city of 15 million people and the army has been ordered to deal with it, and as the general that means you have to decide what to do. You could send in all your troops, but then you would have none spare if there’s another outbreak somewhere else. Instead, you decide to send in a skeleton force of just 1,600 soldiers with orders to recruit members of the public to help them fight the zombies. It’s a novel solution, and leaves you with plenty of men in case there are any other outbreaks, but no one else thinks it’ll work. To prove them wrong, you set out to do the maths. You know that each soldier can hunt down and kill twenty zombies a day. In the evening, each soldier can also recruit one new soldier from the people in the city who will join the fight the following day. However, each night the zombies will fight back and infect five normal people who then become zombies. You know there’s currently 50,000 zombies in the city. Have you made the right decision?

A: Yes, the maths shows this is the right decision. It might take a while, but the strategy will work and the soldiers will regain control of the city.

B: The maths shows that while the strategy is sound, you’d need to send in more troops at the start for it to be successfully implemented.

c: No. The maths shows that this is the wrong decision. No matter how many soldiers you sent in, the strategy will never work. This is because the number of zombies keeps increasing at a rate faster than the soldiers can kill them. The only thing which can stop the outbreak spreading is to nuke the entire city before the situation gets any worse.

Scroll down to see the right answer…

What answer did you get?

A: Your maths must have been wrong. If you send in only 1,600 soldiers, you’ll never managed to get the outbreak under control and the city will be lost within days.
B: Spot on! Just as well you did the maths, or you would have sent in too few troops. In fact, while an initial force of 1,600 men cannot get the outbreak under control by following your strategy, sending in just 67 more in your initial force would ensure it worked.
C: Whoa, I don’t know what happened with your calculations there, but you’re way off. Nuking the city will certainly get the outbreak under control, but it’ll also needlessly kill a lot of innocent people. Maybe you should check your figures before you take quite such a drastic decision.

How to work it out: The maths here is quite complicated, but it reveals something very interesting. To work out the number of zombies at the start of each day, you need to know how many zombies and soldiers there were at the start of the day before. If you know this, you can use the formula Nt+1 = (Nt – (St * 20))*5 to calculate the number of zombies on at the start of any given day. In this formula, St is the number of soldiers at the start of the day before while Nt is the number of zombie at the start of the preceding day. Nt+1 is the number of zombies there will be at the start of the day itself. The value of 20 is the number of zombies which each soldier will kill during each day, while 5 is the number of people which each zombie will infect each night. Using this formula, you can work out what effect sending in different numbers of troops will have. Start by calculating the number of zombies at the start of day two of the campaign (remembering that the number of soldiers will double each day because of the new recruits) and then repeat this for days 3 to 10. If the number of zombies at the start of a day ever becomes zero, then the zombie outbreak will have been extinguished. If it doesn’t, it hasn’t.

If you plug in the initial number of soldiers (1,600) and zombies (50,000) into this formula, you’ll see that the number of zombies quickly spirals out of control, reaching a whopping 17,088,000 by the start of day six, or more than the entire population of the city. This might make it seem like this strategy would never work, but this isn’t true. For example, if you start in 1,700 soldiers and repeat the calculations, you’ll see that the zombie problem gets sorted in just four days. In fact, the difference between success and failure comes down to just one single soldier. If you send in 1,666 soldiers, the city will be over-run by zombies by the start of day nine of your campaign. In contrast, if you send in 1,667 soldiers, the last zombie will be killed on day eight. This means the 1,667th soldier is an example of what Malcolm Gadwell calls a tipping point, where small changes can have big impacts on the final outcome of certain events. It is also an example of a chaotic system where small changes in the starting values can result in very different outcomes further down the road. You can see this in the graph below which shows how the number of zombies changes over time under the four scenarios discussed above.

As shown in this graph, the recruitment problem is a chaotic system, with very different outcomes arising from very similar starting points. In this case, just one soldier makes all the difference between success and failure.

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

21. The Vaccination Program Problem

…because everything’s better with zombies!

Two months ago, a frightening new disease emerged out of nowhere and killed several hundred people in a remote farming community before it was successfully brought under control by the army. Based on the testimony of the few survivors, this disease caused those infected with it to violently attack and bite anyone they could get their hands on. Those who were bitten soon started doing the same and it’s no surprise that this new disease has been dubbed ‘The Zombie Disease’ by news reporters. You’re worried that if a similar outbreak happened in a more heavily populated area, the disease could quickly sweep across the whole country or maybe even the world, before it could be brought under control, and this could bring humanity to an abrupt end.

After many weeks of hard work, you’ve created a virus against this new disease and you’ve calculated that to be effective at stopping any further outbreaks getting out of hand and turning into a full-blown zombie apocalypse, you need to make sure that at least 95% of the population is vaccinated. It takes 6 minutes to give someone a shot and you have a team of 2,500 trained health professionals to administer them, each of whom can work for 16 hours a day. To the nearest whole day, how long will it take to vaccinate enough people in the USA (assuming a population of 250 million) to prevent a zombie apocalypse happening there?

A: 297 days.

B: 396 days.

c: 475 days.

D: 594 days.

Scroll down to see the right answer…

What answer did you get?

A: You’re way off there! You’d twice as many health professionals to get 95% of the population vaccinated in that amount of time.

B: Your team could only get it done that fast if they worked 24 hours a day, and there’s no way they could do that for very long.

C: Something’s gone with your calculations there, or are you only going to give your staff four hours off a night? If they don’t get enough rest they’ll end up making mistakes and you’ll miss your target of having 95% of the population vaccinated.

D: Spot on, but if you’re going to get everyone vaccinated before there’s another outbreak, you’d better get started as soon as possible.

How to work it out: For the vaccination program to be successful, you need to vaccinate 95% of the population, so the first thing you need to do is work out how many people that is. This is done by multiplying 250 million (the total population size) by 0.95 (95% expressed as a decimal fraction). This tells you you’ll need to vaccinate 237.5 million people. Next, you need to work out how long it will take to vaccinate this many people. It takes 6 minutes to administer each vaccine, so the total time is 6 times the number of people to be vaccinated (237.5 million), which is a staggering 1,425 million minutes. However, this isn’t the actual time it will take, because you have 2,500 all of whom can be working at the same time. To work out the actual time, you need to divide the 1,425 million minutes by the number of workers you have on your team (2,500), and that gives you an actual time of 570,000 minutes or, if we divide it by 60 (the number of minutes in an hour), 9,500 hours. Each worker can only work 16 hours a day, so to get the actual number of days it will take, you will need to divide the number of hours required (9,500) by the length of each person’s working day (16 hours), this gives you the required length of your vaccination program in days, which is 593 and three-quarter days, or, to round it up to the nearest whole day, 594 days. As you can see, vaccinating a population against a disease can take a very long time!

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

20. The Containment Zone Problem – Part II

…because everything’s better with zombies!

You have a unit of 600 men under your command. You’ve set up a containment zone surrounding a square of nine city blocks to contain a zombie outbreak, but somehow one of the undead has broken through your line. You give the order for your men to pull back one block. As before, each block is 50 yards long by 50 yards wide and you still need at least two soldiers for every three yards of your perimeter to ensure it’s secure. Do you still have enough men to guard the containment zone once you’ve pulled back, or should you call for reinforcements?

A: With 600 men, I can still maintain a secure containment zone even if we have to pull back.

B: Once we pull back, the perimeter will be too long for my men to guard effectively. I’ll need to call for reinforcements.

Scroll down to see the right answer…

What answer did you get?

A: That’s the wrong choice. While you had more than enough men to secure a 9 city block area, you don’t have enough to hold the larger containment area and because of your decision the city will be lost to the undead.

B: You got it right. You don’t have enough men to secure the larger containment area and you need to call for reinforcements right away!

How to work it out: Again, this is a relatively easy one to work out. First, you need to work out the size of the area you need to secure. Originally, you were securing a square of 9 city blocks. This means it was 3 blocks long by three blocks wide. If your men pull back one block on all sides, this means the area you’ll be trying to secure will be a square of five by five city blocks. As before, this containment zone has four sides, but now each side is five city blocks long, and each block is 50 yards wide. To work out the length of the perimeter, you just need to multiply these three numbers together (50 yards per block by five blocks per side by four sides). This tells you the total perimeter you need to cover is 1,000 yards. Now you the need to work out the total number of men you’d need to adequately cover this larger perimeter. Just as with the smaller perimeter, you need two men ever three yards. This is the same as saying you need 2/3rd of a man per yard, so you divide 1,000 by 3 and the multiply it by 2, giving a total of 667 men (rounded up to the nearest whole man). You only have 600, so now you have to pull back, the only way you can save the city is to call for reinforcements, so you’d better do it right away!

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

19. The Containment Zone Problem – Part I

…because everything’s better with zombies!

There’s been a report of a zombie outbreak on the north side of the city and your unit of 600 men has been sent in to set up a containment zone while others attempt to neutralise the problem. So far the outbreak is limited to a single city block and your containment zone will consist of a one block buffer zone on all sides of the affected area. This means you will have to seal off a square consisting of a total of nine city blocks, each of which is 50 yards long and 50 yards wide. To make sure your defences hold, know you need at least two soldiers for every three yards of your perimeter. You have two choices: set up the containment zone now or wait for reinforcements. If you try to set up the containment zone and you don’t have enough men to man it properly, you risk being over-run and the zombies will take over the city. However, any unnecessarily delay while you wait for reinforcements will give the zombie disease time to spread, making it harder to contain. You’re the one in charge and need to make a decision right now: do you have enough men to set an effective containment zone or should you wait for reinforcements? You have five seconds…

A: Yes, I have more than enough men and the best action is to set up the containment zone right away.

B: I don’t have enough men under my command. It will give the zombie disease time to spread but I need to wait until reinforcements arrive before I can set up an effective containment zone.

Scroll down to see the right answer…

What answer did you get?

A: You made the right decision, with 600 men you have more than enough to station two every three yards around the containment zone’s perimeter.

B: You shouldn’t have waited for reinforcements because you had enough men. This gave the disease time to spread to other city blocks and now you need to set up an even larger containment area. All this because you got your maths wrong!

How to work it out: This is a relatively simple calculation, but the trick is doing in the five seconds you have to make the decision. First, you need to work out the total length of the perimeter of your planned containment zone. The containment zone has four sides, each three city blocks long, and each block is 50 yards long. To work out the length of the perimeter, you just need to multiply these three numbers together (50 yards per block by three blocks per side by four sides). This tells you the total perimeter you need to cover is 600 yards. You the need to work out the total number of men you’d need to adequately cover it. To do this you need two men ever three yards. This is the same as saying you need 2/3rd of a man per yard, so you divide 600 by 3 and the multiply it by 2, giving a total of 400 men. You have 600, so you have more than enough to set up the perimeter right away and without wait for reinforcements.

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

18. The Zombie Horde Problem

…because everything’s better with zombies!

You’re out foraging for food when you turn a corner and find a mass of zombies staggering down the street towards you. Climbing onto a burned out car, you can see that the horde stretches back for five city blocks, each of which is 88 yards long, and that they are moving at 3 miles an hour. You look round for somewhere to hide but the only shelter that’s close enough is an abandoned tank. You climb inside and lock the hatch just as the first of the zombies reach you. If you keep very quiet, they won’t know you’re there and they’ll all walk right passed. If, however, they realise you are there, they’ll surround the tank and you’ll never be able to get out. Being a tank, there’s no way for you to see outside without opening the hatch to take a look, yet night is falling fast and if you stay in the tank too long, you won’t be able to get back to your safe house before it gets dark, so every minute counts. How long will it be before it’s safe for you to open the hatch again? (HINT: There’s 1,760 yards in a mile.)

A: 4 minutes.

B: 5 minutes.

C: 6 minutes.

D: 7 minutes.

Scroll down to see the right answer…

What answer did you get?

A: Oh no! You opened the hatch a minute too soon, meaning there were still zombies all around you. Now they know you’re there, you’ll never get out alive.

B: Spot on! You opened the hatch just as the last zombie has shuffled passed, meaning you can escape back to your safe house before night finally falls.

c: You stayed in the tank a minute too long. You’ll be safe from the zombie horde, but you might not make it back to your safe house before it’s dark.

D: Seven minutes? You’re way out. You’ll never make it back to your safe house in time. Looks like you’ll be stuck in the tank all night.

How to work it out: The first thing you need to work out is how long the horde of zombies is. Each city block is 88 yards in length and the zombies stretch for five blocks so the whole zombie horde is 5*88 yards long. This works out at 440 yards. This means that the last of the zombies are 440 yards away when you close the hatch on the tank. Next, you need to work out how long it will take all the zombies to stagger passed you. To do this, the first thing you need to do is convert the speed from miles an hour to yards a minute. This is done by multiplying the speed in miles an hour (3) by the number of yards in a mile (1,760), to give a speed of 5,280 yards an hour. This number is then divided by the number of minutes in an hour (60) to work out the speed in yards per minute. When you do this, you find out their speed is 88 yards a minute. You can now divide the number of yards the last of the zombies have to travel (440 yards) by this speed (88 yards a minute) to find out that it will take 5 minutes for the last of the zombies to pass your hiding place.

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

17. The Tank vs Motorcycle Problem

…because everything’s better with zombies!

You’ve heard about a safe zone which has been set up and you figure getting there is your best chance of surviving the zombie outbreak in your country. The bad news is that it’s 125 miles away. You have two transport options: a motorbike and a tank. The motorbike‘s much faster and you’ll be able to travel at 60 miles per hour. However, it’s also much more dangerous and there’s a one in six chance you’ll get grabbed by a zombie during each hour you’re on the road. The tank’s a lot slower and can only travel at 7 miles per hour, meaning you’ll be on the road for longer, but it’s also much safer and there’s only a one in fifty chance of a zombie getting you during each hour you are travelling. Which transport option offers you the best chance of getting to the safe zone in one piece?

A: The tank’s slower but safer, so it’s the best option.

B: While the motorbike is less safe, you’ll get there quicker, so overall it’s the best option.

Scroll down to see the right answer…

What answer did you get?

A: Bad choice! There’s a 35.7% chance of you getting caught by a zombie before you reach the safe zone if you choose to travel by tank. This means travelling by tank is marginally more dangerous than travelling by motorbike.

B: Well done, you made the right choice. With a 34.7% chance of getting grabbed by a zombie before you get to the safe zone, the motorbike is safer than the tank.

How to work it out: The key to this problem is working out how long it will take to reach the safe zone using each mode of transport. This is done by dividing the distance you need to travel (125 miles) by the speed of each vehicle. For the tank, this is 125/7 and it means you’ll be on the road for 17.86 hours. For the motorbike, it’s 125/60, meaning you’ll get to the safe zone in 2.08 hours. Now, you can work out the cumulative probability that you’ll get caught by a zombie for each one. For the tank, it’s 1/50 per hour or, if you convert this into a percentage by dividing 1 by 50 and multiplying it by 100, 2%. To get the cumulative probability, you multiply this value by the length of time the journey will take (17.86 hours), which gives you a total chance of falling victim to a zombie before you get to the safe zone of 35.7%. For the motorbike, there’s a 1 in 6 chance of a zombie getting you per hour, or if converted into a percentage, 16.67%. When you multiply this by the time it would take you to get there on the motorbike (2.08 hours) this gives you an overall probability getting killed by a zombie before you get there of 34.7%. Despite the fact there is a far greater risk of you getting caught by a zombie in each hour, the motorbike’s much faster speed means you spend less time on the road, so overall it’s marginally safer.

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.

16. The Wall Problem

…because everything’s better with zombies!

There’s been an outbreak of the zombie disease in Glasgow and Scotland is being over-run. The latest surveillance mission spotted a horde of zombies heading south. They’re currently 125 miles away and are moving at a speed of 3 miles per hour. Scotland is lost and the best way to protect the rest of Britain is to build a defensive barricade on the ruins of the ancient Roman wall which was last used over 1,600 years ago. 750 people can build a one mile long section of ten foot high wall in a day but the wall will need to stretch the entire 73 miles from one side of Britain to the other along the border between Scotland and England if it’s going to keep the zombies out. What’s the minimum number of people you will need to recruit to ensure the wall’s completed before the zombie horde gets to it?

A: 7,884

B: 15,768

C: 31,536

D: 54,750

Scroll down to see the right answer…

What answer did you get?

A: With 7,884 people, you’ll only get 18 and a quarter miles of wall built in time and that will hardly keep the zombies out, will it?

B: With 15,793 people, you’ll only get half the wall built before the zombies get there, and half a wall is little better than no wall at all.

C: That’s right. with 31,536 people you’ll just get the last brick in place as the zombies reach the wall.

D: With 54,750, you’ll get the wall built with plenty of time to spare, but maybe all those extra people could have been doing something else instead.

How to work it out: The first thing you need to work out is how long it will take the zombies to reach you. If you divide the distance they have to travel (125 miles) by the speed they are moving at (3 miles an hour), you’ll find it will take the zombie horde 41.67 hours to reach you. This means you have to have the wall finished in 41.67 hours, or (if we divide this by the number of hours in a day – 24) 1.74 days. Next, you need to work out how many people you would need to finish the wall in this time. 750 people can build one mile of wall in a day, but the wall needs to be 73 miles long. If you multiply these two numbers together, you’ll find that 54,650 people could build the whole wall in one day. Except you have 1.74 days and not just one day, so you need to divide this number by 1.74 to get the number you need to complete the wall before the zombies get there, and this is 31,536 people. That’s a lot of people, so you’d better start recruiting them right away!

While the problems provided here are copyright of Maths With Zombies, if you are a teacher, you can use any of these problems for free in your classes – but please credit Maths With Zombies as the original source (e.g. Downloaded from MathsWithZombies.wordpress.com). You can download a PDF handout of this problem from here.

If you do use this problem in a class, please post a comment here to let me know how you used it and how it was received by your students. These problems cannot be used for any commercial purpose without express written permission.

*****************************************************************************
From the author of For Those In Peril On The Sea, a tale of post-apocalyptic survival in a world where zombie-like infected rule the land and all the last few human survivors can do is stay on their boats and try to survive. Now available in print and as a Kindle ebook. Click here or visit www.forthoseinperil.net to find out more. To download a preview of the first three chapters, click here.

To read the Foreword Clarion Review of For Those In Peril On The Sea (where it scored five stars out of five) click here.